Here We have given NCERT Solutions for Class 9 Maths Chapter 1 Number System. These solutions are posted very carefully without any errors.
Your 9th standard is a major stepping point in your schooling because you are made aware of the looming 10th board exams at the end of two years. The foundation of all the relevant knowledge of mathematics during these two years is laid right here with Chapter 1 – Number Systems.
Table of Contents
NCERT Solutions for Class 9 Maths Chapter 1 Number System Exercise 1.1


NCERT Solutions for Class 9 Maths Chapter 1 Number System Exercise 1.2


NCERT Solutions for Class 9 Maths Chapter 1 Number System Exercise 1.3





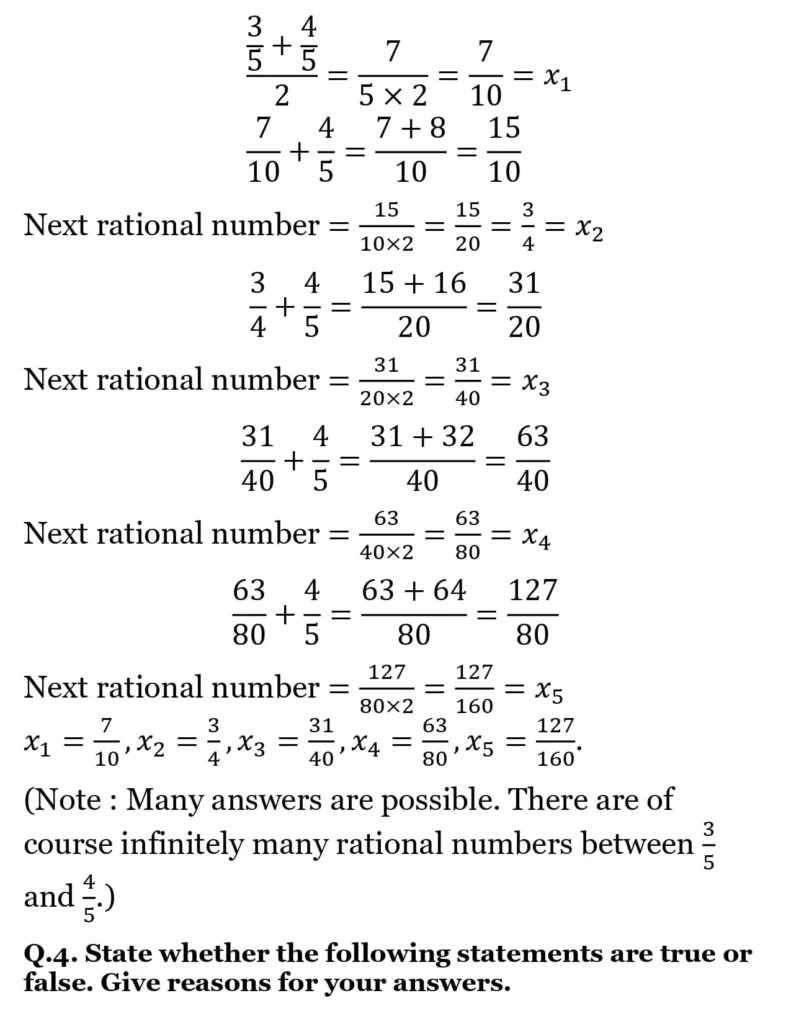
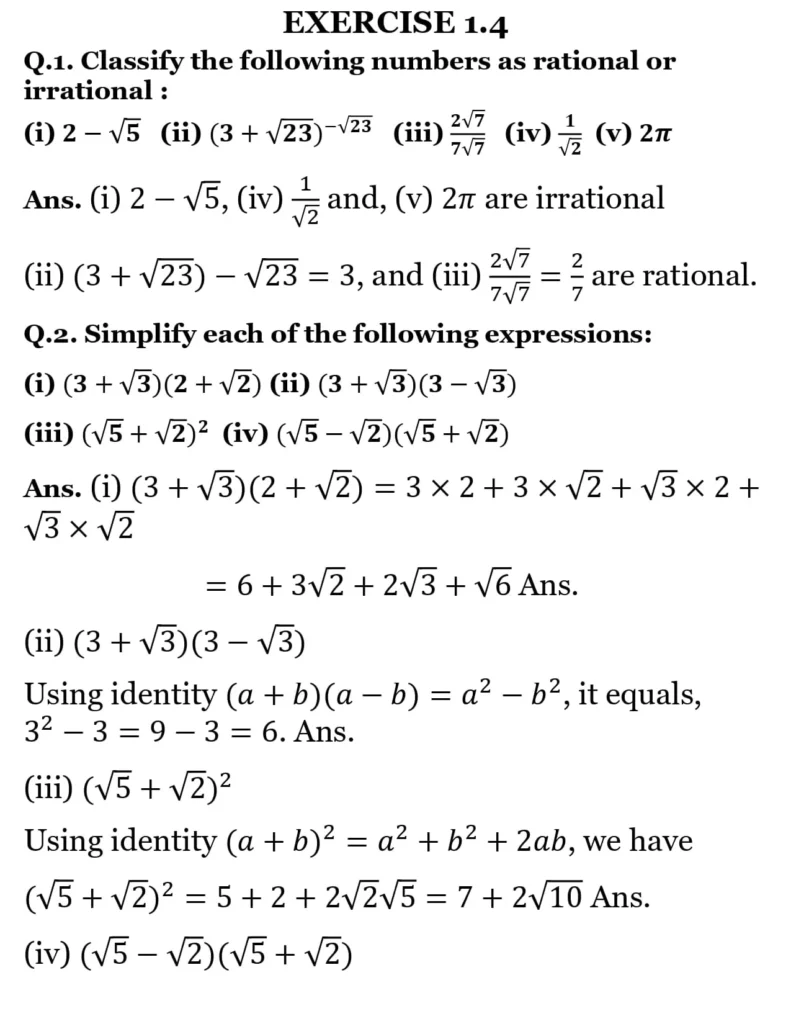
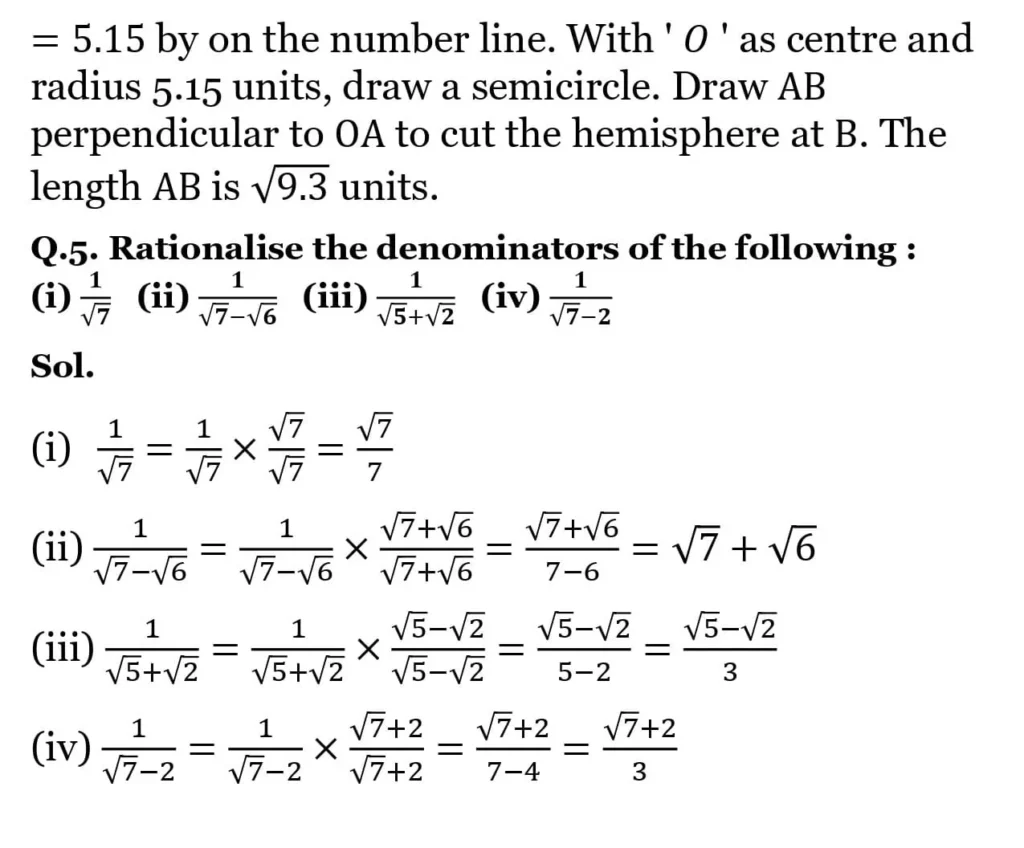
NCERT Solutions for Class 9 Maths Chapter 1 Number System Exercise 1.4

NCERT Solutions for Class 9 Maths Chapter 1 Number System Exercise 1.5
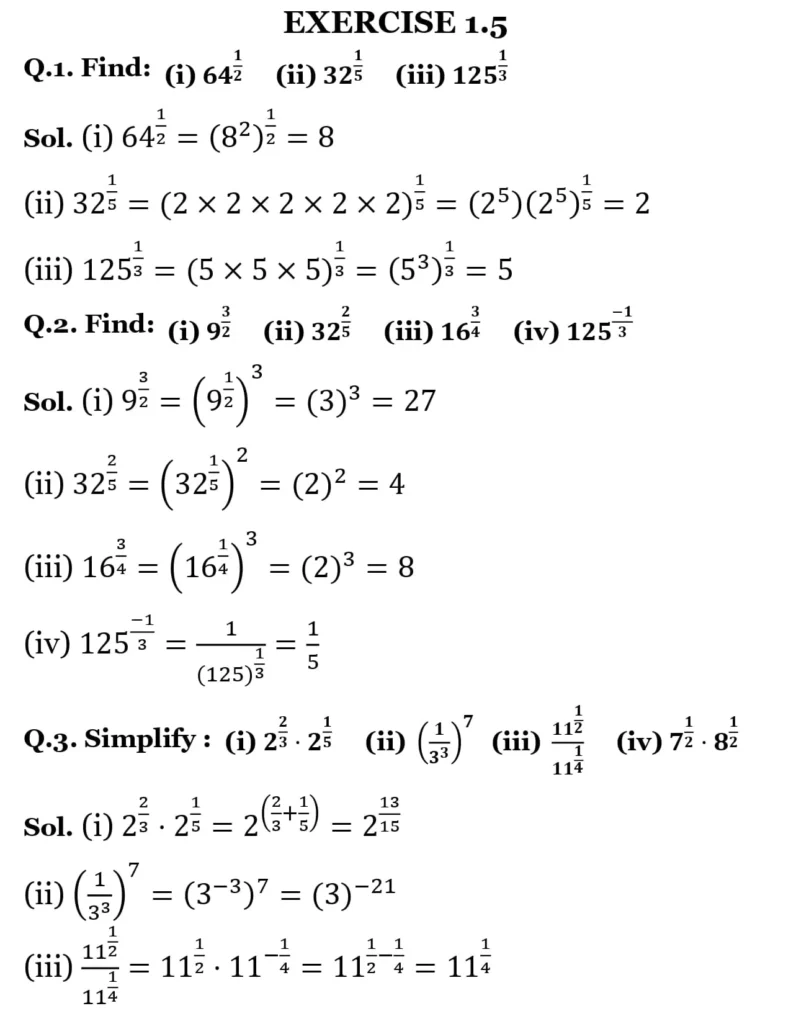

Check:- NCERT Solutions for Class 9 Maths
Types of Number Systems in Chapter 1
- Rational numbers (denoted by r): Numbers that can be written as p/q where q is a non-zero number. This category includes all integers, natural numbers and whole numbers within it. These can be represented as either a fraction or decimals. Examples include 1 /3, 0.25, 100. 10
- Irrational numbers (denoted by s): These numbers cannot be expressed in the form of p/q where q is a non-zero number. Examples include π,√2, √7 etc.
- Real numbers: The combination of all rational and irrational numbers gives the set of all real numbers. This term also implies the existence of imaginary numbers and that is a more complex topic that you will get into during your higher studies
Decimal expansion of rational numbers
While the definition of rational numbers given above uses the fraction form of the number, it is natural that we can express them as decimals as well. In this way, there are two types of expansions that you will encounter in the exercises of NCERT Class 9 Maths chapter 1.
- Terminating: The decimal values go to a certain number of digits meaning that the remainder while dividing the fraction is 0. For example, ⅖ is 0.4, it terminates at this point
- Non-terminating: The values after the decimal keep on going without any limit for example ⅓ is 1.33333….., in such cases, the recurring portion of the decimal has a bar drawn over it to signify that it is recurring.
- Both terminating and non-terminating recurring decimal expansions are possible with rational numbers. However, for irrational numbers, the pattern will be non-terminating and non-recurring. For instance pi – which has a non-recurring pattern that goes on to infinity.
Operations on real numbers
By performing operations on irrational numbers you may get a rational number as a result – for example √7 x √7 = 7, a rational number that is the product of two irrational numbers. However, not every operation leads to a rational number coming from the result.
- The sum/difference between a rational and an irrational number is irrational
- The product/quotient of a rational number (except zero) and an irrational number are irrational
- Any operation on two irrational numbers may give an irrational number or a rational number.
FAQs (Frequently Asked Questions)
Why are Class 9 Maths NCERT Solutions Chapter 1 Important?
Understanding number systems is the basis of being able to effectively do algebra; if you cannot understand the terms and types of numbers you will encounter in practically every subsequent chapter then you will not be able to attempt any part of them. These various types include rational numbers, irrational numbers, integers, whole numbers, and natural numbers, among others. In order to get a brief overview of the chapter, including definitions for the types of natural numbers given in Class 9 Maths chapter 1 solutions keep reading on ahead.
Do I Need to Practice all Questions Provided in NCERT Solutions Class 9 Maths Number Systems?
NCERT class 9 chapter 1 solutions contain the answers to 5 exercises (1.1 to 1.5) which contain several questions each. By going through these questions and their solutions you will become very thorough with the concepts such as giving the decimal expansions for fractions by long division, simplifying expressions containing irrational numbers, practising the laws of exponents and representing figures on a number line. You should also be familiar with the concepts of identities relating to square roots and exponents.
Class 9 maths NCERT solutions for Number Systems can be found on our site and recommend going through them carefully to make yourself more capable of handling every chapter in the NCERT maths curriculum.
Tagged with: 9 class maths chapter 1 | 9th class math chapter 1 question answer | cbse 9th class maths chapter 1 | cbse class 9 maths chapter 1 solutions | cbse class 9th maths chapter 1 | ch 1 class 9th maths | chapter 1 maths class 9 ncert solutions | class 9 ch 1 maths ncert solutions | class 9 maths chapter 1 ncert
